This post is an excerpt from the journal ISA Transactions. All ISA Transactions articles are free to ISA members, or can be purchased from Elsevier Press.

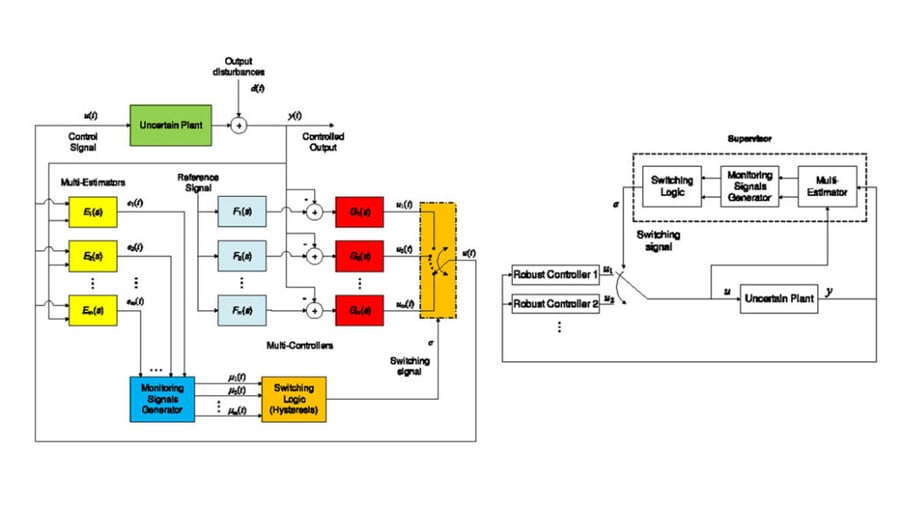
Abstract: In this paper, the design of decentralized switching control for uncertain multivariable plants is considered. In the proposed strategy, the uncertainty region is divided into smaller regions with a nominal model and specific control structure. The underlying design is based on the quantitative feedback theory (QFT). It is assumed that a MIMO-QFT controller exists for robust stability and performance of the individual uncertain sets. The proposed control structure is made up by these local decentralized controllers, which commute among themselves in accordance with the decision of a high level decision maker called the supervisor. The supervisor makes the decision by comparing the local models’ behaviors with the one of the plant and selects the controller corresponding to the best fitted model. A hysteresis switching logic is used to slow down the switching to guarantee the overall closed loop stability. It is shown that this strategy provides a stable and robust adaptive controller to deal with complex multivariable plants with input–output pairing changes during the plant operation, which can facilitate the development of a reconfigurable decentralized control. Also, the multirealization technique is used to implement a family of controllers to achieve bumpless transfer. Simulation results are employed to show the effectiveness of the proposed method.

Free Bonus! To read the full version of this ISA Transactions article, click here.

Enjoy this technical resource article? Join ISA and get free access to all ISA Transactions articles as well as a wealth of other technical content, plus professional networking and discounts on technical training, books, conferences, and professional certification.

Click here to join ... learn, advance, succeed!

2006-2018 Elsevier Science Ltd. All rights reserved.